(iv) The Six Canons of Hindu Architecture (Āyādi-ṣaḍvarga)

According to the Samarāṅgaṇasūtradhāra the Āyādi is a group of six, namely Aya [Āya?], Vyaya, Aṃśa, Ṛkṣā, Yoni, and Vāra-tithi. It is said these are the six formulas, “with which the perimeter of structure should conform”. They are in a way “six proportions, six main component parts of a building comprising Adhiṣṭhāna (base), Pāda or Stambha (column), Prastara (entablature), Karṇa (ear i.e. wings), Śikhara (roof) and Stūpi (dome)”—Ency. H. A. p. 500.

These formulae in different texts are not the same. According to the Samarāṅgaṇasūtradhāra they are Āya, Vyaya, Yoni, Tārā, Bhavanāṃśaka and Gṛhanāma. Āya represents the group of eight beginning with Dhvaja, Dhūma, Siṃha, Śvā, Vṛṣa, Khara, Kuñjara and Dhvāṅkṣa. It also lays down the respective efficacy of these Āyas in the respective planning together with their virtues and defects (vv. 21-24).

The Vyaya represents the group of three—Piśāca, Rākṣasa and Yakṣa. Similarly the Aṃsas are also three—Indra, Yama and Rājā. As regards the Ṛkṣā, Tārā, they are distributed in three groups of nine each—Sura-Gaṇa, Rākṣasa-Gaṇa and Mānuṣa-Gaṇa. These 27 Tārās are of common knowledge.

This Chapter gives a detailed information on the application of these sixfold Karaṇa in the house operations. It says, “With the group of three in favour, the operations are auspicious, with two or one, inauspicious and on the other hand, with four it is very much auspicious”.

In the end this Chapter also takes up another set of six technically called Chandas. They are Meru, Khaṇḍa Meru, Patākā, Sūcī, Uddiṣṭa and Naṣṭa, a detailed notice of which forms the subject-matter of the last section.

All these Canons are inter-related: Whether the orientation of a building is correct and whether the measurements as laid down in regard to the different structures are correct, auspicious and conform to the orientation, are further tested by this very fundamental canon of the Āyādi six-formulas. Saḍvarga [Ṣaḍvarga], accordingly is a group of six, six formulas, with which the perimeter of a structure should confirm, six proportions, six main component parts of a building comprising adhiṣṭhāna (base), pāda or stambha (column), prastara (entablature), (Karṇa ear, wings), śikhara (roof) and stūpi (dome). This is only a general definition of the Saḍvarga [Ṣaḍvarga]. According to the Mānasāra the Āyādi-ṣaḍ-varga, however, represents a set of six formulas with which any particular measurement must conform before it can be accepted.

Thus our previous thesis that this canon is a step forward towards the correct orientation and the proportions of measurements of a structure is supported.

‘The invention of Āyādivarga is an architectural device, the intention of which is to find out a proper orientation to the structure and a proper dimension Among the architectural conventions of India, Āyādivarga occupies an important place. Every measurement before it is accepted is required to satisfy these six fundamental requisites. Every architectural treatise prescribes a variety of dimensions, but these have to be further subjected to an examination in view of the fact that Hindu structures have to satisfy the considerations of auspiciousness, propriety and orientation. It was with a view to enabling the builder to select the auspicious and proper measurement that these traditional architectural formulas known as Āyādiṣaḍvarga are described in Indian architectural literature.’

How the correct orientation of a building particularly of a temple is dependent on these formulas, can be understood with especial reference to Yoni, one of the six members of this ‘varga’ (the others being Āya, Vyaya, Ṛkṣā, Tithi and Vāra). According to our tradition, orientation according to its location on Īśa is a pre-requisite of planning. Thus temples possess either the Dhvaja or the Vṛṣa Yoni (the Yoni is eightfold—the other six are Vāyas, Dhūma, Siṃha, Śvā, Vānara and Gaja, which also determine the eight Vāstupuruṣas as we shall soon see). Mallaya rightly interprets this tradition when he says—“Temples located in any of the quarters beginning with Īśa and ending with Yama in a village, capital or city will have Dhvaja Yoni (i.e. Yoni No. 1). In the remaining quarters, the Yoni will be Vṛṣa, (i.e. No. 5). The image that is to be installed will possess the Yoni of the temples. Thus an image enshrined in a temple which faces the west will have Yoni No. 1, because the Yoni of the temple which faces the west is Yoni No, 1. The idea in brief is that the sanctum and the image will possess similar Yoni and facing”.

Thus of all the Āyādi-vargas, Yoni is regarded as the most important. Accordingly it has claimed the greatest attention at the hands of every authority on architecture. As already referred to, Yonis are eight in number which correspond to the eight quarters and hence their importance in the determination of the exact orientation is understandable. They are sometimes refered to by numbers 1, 2, 3, 4, 5, 6, 7 and 8. Dhvaja Yoni is accordingly called Yoni No. 1 and is positioned in the due East. Dhūma Yoni, is Yoni No. 2 and is located in the South-East. Siṃha Yoni is Yoni No. 3 and is located in due South and so on. Dr. Kramrisch completes this thesis of imperative importance of Yoni formula in relation to the orientation of a building (vide her Hindu Temple pp. 37-9) and her learned observations are worth quoting: “The position and orientation of a temple and of any building are determined analogous to the method according to which the place of sun or moon or one of the planets is found in the circle of Nakṣatras. According to the ‘Sūryaprajñapti’ the longitude of the heavenly body expressed in minutes is to be divided by 800, the quotient shows the number of Nakṣatras through which the planet has already passed and the remainder, the traversed part of the Nakṣastra in which it is at the time. In a similar way is ascertained the position of a building in the cosmos; that is the direction which it is to face: the measurement of the building is to be divided by 8. The remainder indicates the particular direction which will be its own out of the 8 directions. This particular direction is the Yoni, its birthplace in the cosmos, where it is marked by its Vāstupuruṣas.

The Vāstupuruṣas, and the respective remainders are given by Utpala commenting on the ‘Bṛhat-Saṃhitā’ Ch. LIL 73, according to the teaching of other Ācāryas, and not of Varāhamihira.

“If the remainder is 1, then the yoni is Dhvaja, and the building faces East, if the remainder is 2, the yoni is the S E and so forth. If there is no remainder the building would have to face North-East. This is to be avoided by all means; it would be of evil portent were the building to face any of the corners of the square;similarly also the remainder should not be 2, 4 or 6, it must be uneven, so that the entrance of the building faces the East, preferably or also the West and less readily the North and still permissibly, the South The remainder is found in different texts by taking account of various measures of the building to be set up. The perimeter, for instance is multiplied by 3 and divided by 8. The remainder is that of yoni; should there be no remainder, the perimeter and proportions of the intended structure have to be altered. Death, destruction and varied ills result from a wrong orientation. If the building were to obstruct the course and order of cosmos it would provoke disorder in the kingdom, and in the body of the builder. Yoni is an architectural formula, the remainder gained through it, assures the fitness of the structure in order of things and the well being of the builder and his surroundings The remainder, particularly, is however, the Vastu, itself.”

Yoni constitutes the life and breath of the structure, particularly of a house. A proper and auspicious yoni is therefore required to be chosen for the same.

Thus the Manuṣyālayacandrika observes:

yoniḥ prāṇā eva dhānmāṃ yadasmād |
grāhyastattadyogyayoniprabhedaḥ |

Considering the fruits thereof, the general rule is that all odd yonis are good and even ones bad. Accordingly, ‘Dhvaja’ ‘Siṃha’ ‘Vṛṣa’ and Gaja are auspicious and ‘Dhūma’, ‘Kukkura’, ‘Khara’ and ‘Vāyasa’ are inauspicicus. The Śilparatna furnishes the details concerning the fruits that particular Yonis are supposed to yield. There is a consensus of opinion that Dhvaja is the best of all the auspicious yonis (cf. Mallaya).

With this general introduction and the utility of these formulas especially the yoni one in regard to the orientation of a building let us expound this canon in further details. It may be remarked that the different texts take different criterion of multiplication to obtain the yoni or the gain or loss as would be evident below: Utpala’s commentary, ‘Bṛhat-Saṃhitā’ ch. LII. 73 multiplies the interior length and breadth of the building and divides it by 8. The remainder is the Yoni. Similarly ‘Vāstu-rājavallabha’, ch. III. 8 enjoins. In other texts, the height of the temple is taken into account (Vaikhānasāgama, ch. VI) while the ‘Tantra-sammucchaya [Tantrasamuccaya?]’, Part I. Ch. II. 3, considers the perimeter, and the Mānasāra, ch. IX. 68-74, the breadth of the building. Perimeter or breadth are multiplied by 3; this indicates an area equalling that of a circle with the breadth or the perimeter of the building as its diameter”.

So much about the Yoni formula The yoni is but one of the six formulae, the Āyadi-ṣaḍ-varga—Āya, Vyaya, Ṛkṣa, Yoni, Tithi and Vāra—and to this group, of six is also added the formula of Vayas.

In the Saḍvarga [Ṣaḍvarga], the remainder determines, the gain or loss which will accrue to the builder, the Nakṣatra (ṛkṣā), the lunar day (tithi) and the solar day (vāra) on which it is good to build that particular building. Though these formulae have their special domain of astrology in general, they are applied to building as though it is a living entity whose destiny is to be determined.

Now avoiding other details let us reproduce these formulae. As already remarked, different texts treat this canon differently. There are however principally two traditions, one represented by the Mānasāra and the other by the texts like, Kāśyapa-śilpa, Śilparatna, Vāstuvidyā, Manuṣyālaya-Gandrikā and the Samarāṅgaṇasūtradhāra In the former the length or breadth or circumference is to be multiplied and then divided while in the latter it is the perimeter which is to be so multiplied and then divided. The following reproductions from Mallaya (cf. Studies in Sanskrit texts on Temple Architecture) will make the whole position clear:

Mānasāra Formula:

Here L=Length; B=Breadth; C=Circumference.

Formula of the other texts:

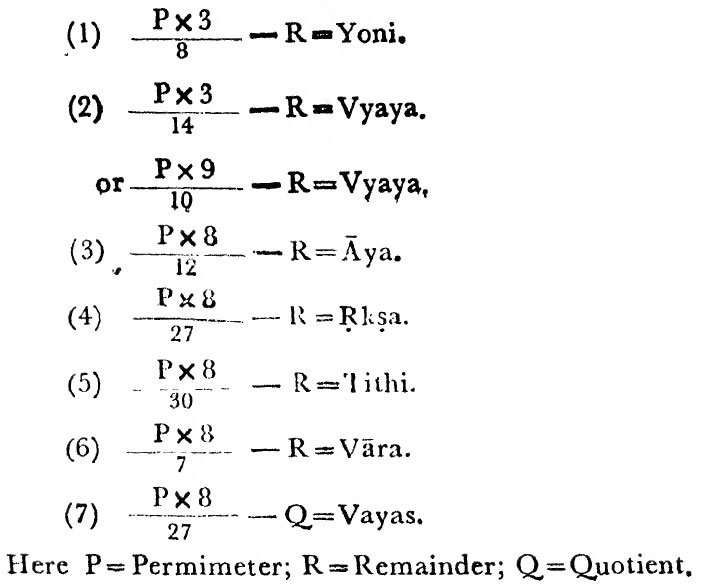
Here P = Permimeter; R = Remainder; Quotient,

“Each formula is a Śāstrika technicality and is known after the divisor in each case which is a figure corresponding to the number that forms each well-known group. Yoni thus represents the group of eight, viz., Dhvaja, Dhūma, Siṃha, Kukkura, Vṛṣa, Khara, Gaja and Vāyasa. Āya stands for the group of twelve commencing with Siddhi and Vyaya for the group of twelve beginning with ‘Sikhara [Śikhara]’. ‘Ṛkṣa’ represents the group of 27 Nakṣatras beginning with Aśvinī, ‘Tithi’ the group of 30 lunar days starting with Prathamā (of both the Full and the New Moon) and Vāra the group of 7 days of the week, beginning with Arka (Sunday). Thus the figures employed as divisor are clear enough, but concerning the multiplicatory numbers as 3, 8, etc., nothing is known.”

The application of the different formulae may be illustrated with reference to 3 cubits measure type as follows (cf. Mallaya):

The perimeter of a structure having 2¾ cubits breadth is 11 cubits. Here yoni is No. 1.

Much has been said on the importance of these formulas in relation to the correct orientation of the building. They are equally important in relation to the correct and apt measurement to which a particular building should conform. They are applied in measuring both the architectural and the sculptural objects. According to the Mānasāra the measurement of length is tested by the formulas under Āya and Ṛkṣa, of breadth under Vyaya and Yoni and of circumference or height under Vāra and Tithi.

Dr. Acharya, therefore, rightly observes on the importance of these formulas in helping to arrive at correct measurements:

‘The necessity of these Saḍ-varga formulas seems due to the fact that in most instances where the measurement of any object is concerned, the Āgamas, the Bimbamāna, the Mānasāra and the other works on architecture quoted more dimensions than one. Thus for the length that an object is to be of, they instead of giving a single figure would quote, say, nine different measures. The Mānasāra in fact invariably gives nine different lengths, nine different breadths, and five heights concerning a building or image. Out of these different and varying measures which is to be selected would be determined by the application of the six formulas. Any of the different measures prescribed is open to be accepted only when it satisfies the tests of the Saḍ-varga. By a verification of the measurements with the respective formulas it would eliminate the risk of dimensions being selected that would be disproportionate among themselves and improper. This might have been the purpose that the authorities had in contemplation in prescribing the rules of the Āyādi Saḍ-varga [Ṣaḍvarga?] in all the architectural treatises. The testing of measurements by the Saḍ-varga [Ṣaḍ-varga?] thus formed one of the most important points to be followed in architecture and sculpture, and we find a reference to it in these ancient works so many times and almost without exception wherever there are any specifications prescribed’.

Mr. V. K. R. Menon has worked these formulas to their most logical conclusions, though all his conclusions cannot be accepted without some modifications—vide his pamphlet ‘Six Canons of Indian Architecture’—the details of which may be avoided here and the reader is referred to Dr. Acharya’s Encyclopaedia for some of his conclusions with comments of the learned author of the Encyclopaedia (see page 509)